Calculating margin on sales is an important part of business. You need to know:
- What variable costs you need to cover for each sale. The concept is the same whether you’re selling products or services.
- Given the selling price and the variable costs covered, what the contribution / margin of each sale is.
- Given the contribution of each sale, how many sales are then needed to cover your fixed costs (the costs you incur regardless of any other activity in your business) in order to be profitable or cash flow positive, depending on whether you are looking at profit or cash.
The common error
Margin is commonly referred to when talking about “how much are we making on this…”. Then the conversation turns to mark-up, which is simply what we add to our costs in order to get the selling price needed (assuming the market will tolerate that price) to generate the needed / desired margin. This is all well and good, but the problem arises when people start equating the concepts of “mark-up” and “margin”. The most common error made, even by people in sales, is to talk about mark-up as if it was margin. Thus:
“…we want to make x% margin, so we’ll mark it up by x%.”
Not only is this wrong, but the bigger the margin / mark-up we’re talking about the bigger the losses you’ll make in dollar terms. Let’s see the impact with a couple of simple examples. We’ll assume we have a cost base of $20.
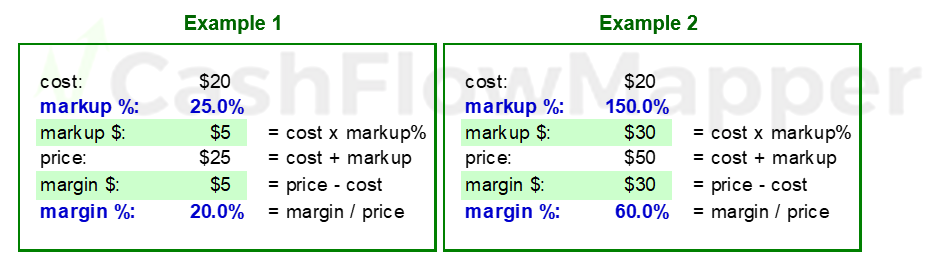
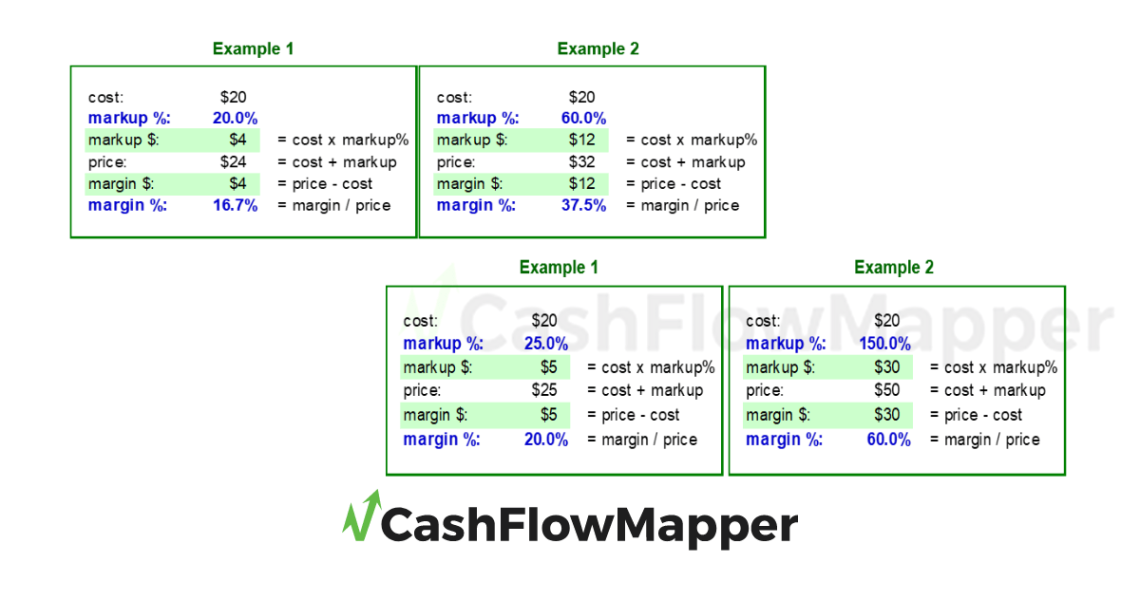
In both examples, notice that the $ value of the margin and mark-up are the same (green highlighted sections), but as we seek higher margins in percentage, the required mark-up percentage increases markedly. In Example 1, marking up by 25% is needed to give us 20% margin. The disparity between mark-up and margin percentages is shown in Example 2 where 150% mark-up is required to earn 60% margin on the sale.

The implications of getting it wrong
The consequences of equating margin and mark-up percentages is clear, particularly if you know what your required margin needs to be. Let’s see the margin we actually make under both examples by marking up our cost by what we wrongly think is also the margin we’ll make.
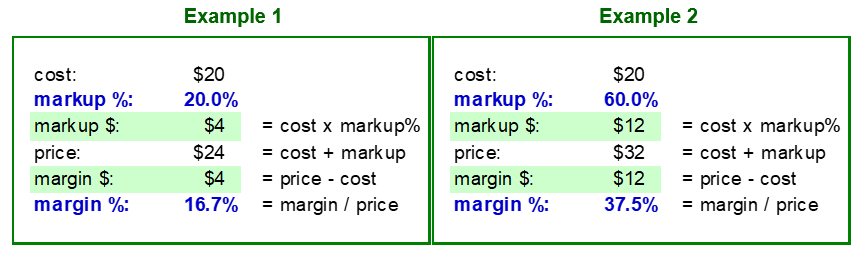
In Example 1, with a 20% mark-up, we earn 16.7% margin, not 20%. We lose $1 margin per unit, which will add up. This is a 20% loss in margin.
In example 2, the error is even more costly. Marking up by 60% earns us 37.5% margin, or $18 less per unit – a 60% loss in margin.
Notice that the error results in a loss of margin in percentage terms equal to the mark-up – just to rub it in!
To sum up, we need to be clear whether we are talking numbers or percentages when talking about margin and mark-up. The numbers are the same, the percentages aren’t.
A greater understanding
The above is all you need to know regarding the differences between margin and mark-up. Following is the mathematical relationship, using Example 1 data to illustrate.
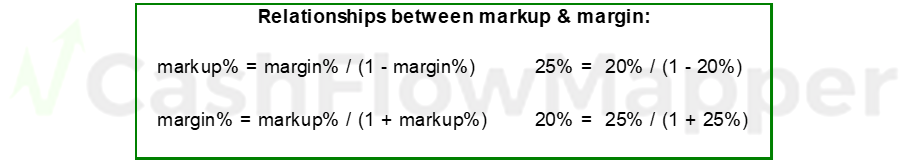
Other useful relationships
At times, we have certain information available to us in the margin equation, and we need to calculate the information we don’t have. It’s also good practice to see what impact differing price, cost and margin scenarios have on our budgeting and forecasting too. Again, Example 1 data is used.